Diagonal Difference Hackerrank Solution in C & C++

In this article, We are providing Diagonal Difference Hackerrank Solution in C, C++, and Java programming Languages. This programming problem belongs to hackerrank 30 days of code, and we are going to find the Hackerrank Diagonal Difference Solution in C. We have to find the Diagonal Difference of an N*N matrix or a square matrix. We have to find the right diagonal sum of a matrix and the left diagonal sum of the matrix, then find out the hackerrank diagonal difference of a matrix. In this matrix, we already know that our matrix size is an N*N.

Diagonal Difference Hackerrank

Function Description
Input Format
Constraints
Output Format
Sample Input
Sample Output
Explanation of Diagonal Difference Hackerrank
The Logic of the Diagonal Difference Hackerrank
Diagonal Difference Hackerrank Solution in C
Diagonal Difference Hackerrank Solution in C++

Given a square matrix, calculate the absolute difference between the sums of its Diagonal Difference Hackerrank Solution.

For example, the square matrix arr is shown below:

1 2 3
4 5 6
9 8 9

The left-to-right diagonal = 1 + 5 + 9 = 15 . The right to left diagonal = 3 + 5 + 9 = 17 . Their absolute difference is |15-17| = 2.

Function Description

Complete the DiagonalDifference function in the editor below. It must return an integer representing the absolute diagonal difference.

diagonalDifference takes the following parameter:

arr: an array of integers.

Input Format

The first line contains a single integer, n, the number of rows and columns in the matrix arr. Each of the next n lines describes a row, arr[i], and consists of n space-separated integers arr[i][j].

Constraints

-100<=arr[i][j]<=100

Output Format

Print the absolute difference between the sums of the matrix's two diagonals as a single integer.

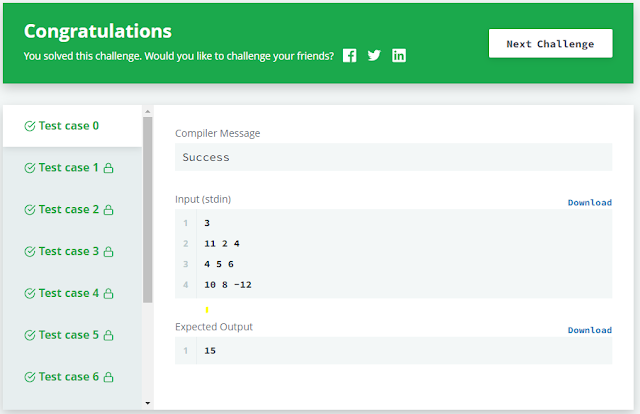
Sample Input

3
11 2 4
4 5 6
10 8 -12

Sample Output

Explanation of Diagonal Difference Hackerrank Sample

The primary diagonal is:

Sum across the primary diagonal: 11 + 5 - 12 = 4

The secondary diagonal is

Sum across the secondary diagonal: 4 + 5 + 10 = 19

Difference: |4 - 19| = 15

Now the last step is to find the difference between the sum of diagonals, so add the first diagonal and the second diagonal after that mod the difference so | 4 - 19| = 15. Hence we got our solution.

Note: |x| is the absolute value of x

Check This- Hackerrank 30 days of code Solution.

Diagonal Difference Hackerrank Logic

So the logic is straightforward in this diagonal difference hackerrank problem. We have to find the diagonal sum of the matrix, and after seeing the total amount. We have to find out the difference between both diagonal sums. If the difference of both diagonal matrices is negative, then find the Mod or, in the end, print the output. Get the Hackerrank Diagonal Difference Solution in C language See the above logic solution with an example in the explanation.

Submit your solution here:-Click here

Diagonal Difference Hackerrank Solution in C

#include <math.h>
#include <stdio.h>
#include <string.h>
#include <stdlib.h>
#include <assert.h>
#include <limits.h>
#include <stdbool.h>
int main()
{
 
    int n, j;
    int i=0,RightDiagonalSum=0,LeftDiagonalSum=0, firstarray, secondarray;
    
 scanf("%d",&n);
    int a[n][n];
    
 for(int firstarray = 0; firstarray < n; firstarray++)
 {
       for(int secondarray = 0; secondarray < n; secondarray++)
    {
         
          scanf("%d",&a[firstarray][secondarray]);
       }
    }
 
  while(i<n)
  {
    RightDiagonalSum=RightDiagonalSum+a[i][i];
    i++;
  }
    
 j=n-1,i=0;
    
  while(i<n)
  {
    LeftDiagonalSum=LeftDiagonalSum+a[i][j];
    i++;
    j--;
  }
    
 printf("%d",abs(RightDiagonalSum-LeftDiagonalSum));
    return 0;
}

Diagonal Difference Hackerrank Output

So as we already know, what is the logic,, so without wasting time, let's just implement the above logic in our diagonal difference hackerrank problem with an example? Take a MATRIX with the same number of rows and columns. Below is the Hackerrank Diagonal Difference Solution in C++ Programming language.

Diagonal Difference Hackerrank Solution in C++

#include <iostream>
using namespace std;
int main() 
{
  int N, LeftDiagonalSum = 0, RightDiagonal = 0;
  cin >> N;
  int a[N][N];
  for (int i = 0; i < N; i++) 
  {
    for (int j = 0; j < N; j++) 
    {
      cin >> a[i][j];
      if (i == j) 
   {
        LeftDiagonalSum = LeftDiagonalSum + a[i][j];
      }
    }
  }
  
  for (int i = 0; i < N; i++) 
  {
    for (int j = N - 1 - i; j >= 0;) 
    {
      RightDiagonal = RightDiagonal + a[i][j];
      break;
    }
  }
  cout << abs(LeftDiagonalSum - RightDiagonal) << endl;
  return 0;
}